The Value of Stochastic Blockmodels in Social Network Analysis
Practical Solutions and Value
The use of relational data in social science has surged over the past two decades, driven by interest in network structures and their behavioral implications. However, the methods for analyzing such data are underdeveloped, leading to ad hoc, nonreplicable research and hindering the development of robust theories.
Two emerging approaches, blockmodels and stochastic models for digraphs, offer promising solutions. Combining these approaches could address their limitations and enhance relational data analysis.
Advancing Social Network Analysis
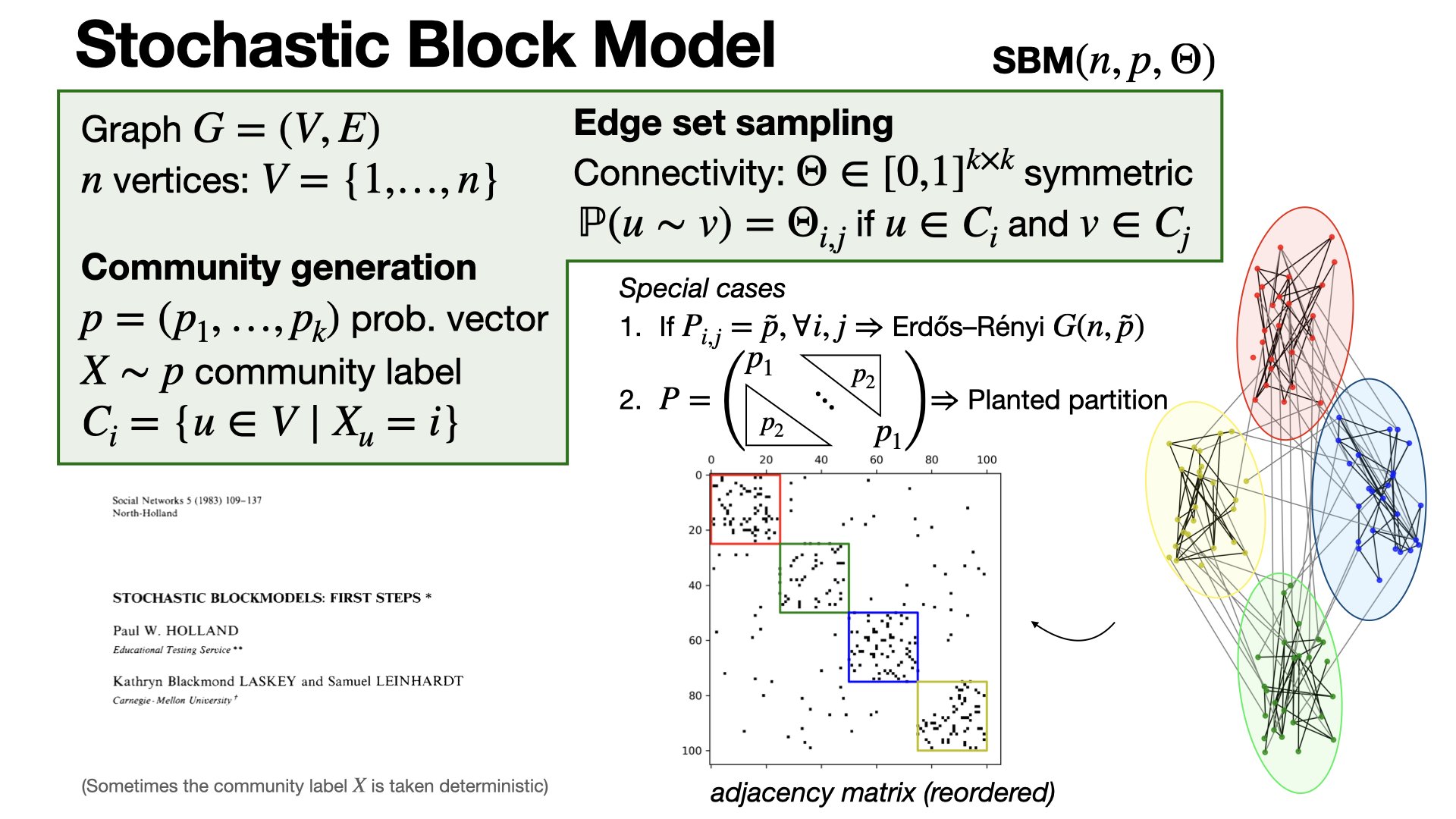
Educational Testing Service and Carnegie-Mellon University researchers propose a stochastic model for social networks, partitioning actors into subgroups called blocks. This model extends traditional block models by incorporating stochastic elements and estimation techniques for single-relation networks with predefined blocks. The study discusses a merger of this approach with stochastic multigraphs and blockmodels, describes formal fit tests, and uses a numerical example from social network literature to illustrate the methods.
Practical Applications
A stochastic blockmodel is a framework used for analyzing sociometric data where a network is divided into subgroups or blocks, and the distribution of ties between nodes depends on these blocks. In practical applications, stochastic blockmodels analyze single-relation sociometric data with predefined blocks, simplifying estimation by focusing on block densities the probability of a tie between nodes in specific blocks.
Pair-Level Structures in Social Networks
The study explores advanced blockmodeling techniques to analyze reciprocity and pair-level structures in social networks. It discusses the concept of reciprocity and introduces the Pair-Dependent Stochastic Blockmodel (PSB) and the Stochastic Blockmodel with Reciprocity (SBR), which includes parameters for mutual, asymmetric, and null ties. The text also covers estimation using Maximum Likelihood Estimation (MLE) and model fit testing.
Bayesian Approaches
The excerpt addresses the closure challenge and explores the Bayesian approach to generating blocks, where blocks are not predetermined but discovered from data. This approach specifies the number of blocks, block size distributions, and density parameters for different block types. The Bayesian model allows for posterior probability estimation of block memberships, aiding in a more systematic relational data analysis.
Evolve Your Company with AI
If you want to evolve your company with AI, stay competitive, use for your advantage Advancing Social Network Analysis: Integrating Stochastic Blockmodels, Reciprocity, and Bayesian Approaches.
Discover how AI can redefine your way of work. Identify Automation Opportunities, Define KPIs, Select an AI Solution, and Implement Gradually. For AI KPI management advice, connect with us at hello@itinai.com. And for continuous insights into leveraging AI, stay tuned on our Telegram t.me/itinainews or Twitter @itinaicom.
Discover how AI can redefine your sales processes and customer engagement. Explore solutions at itinai.com.

























